
How To Graph Reflections Across Axes The Origin And Line Y X Video Lesson Transcript Study Com
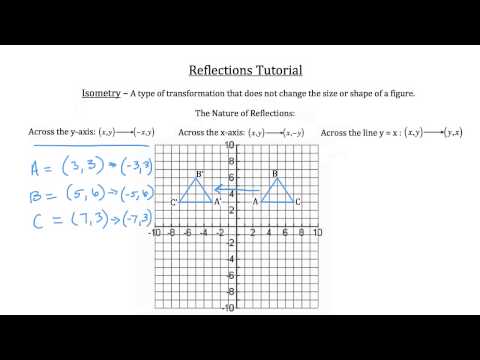
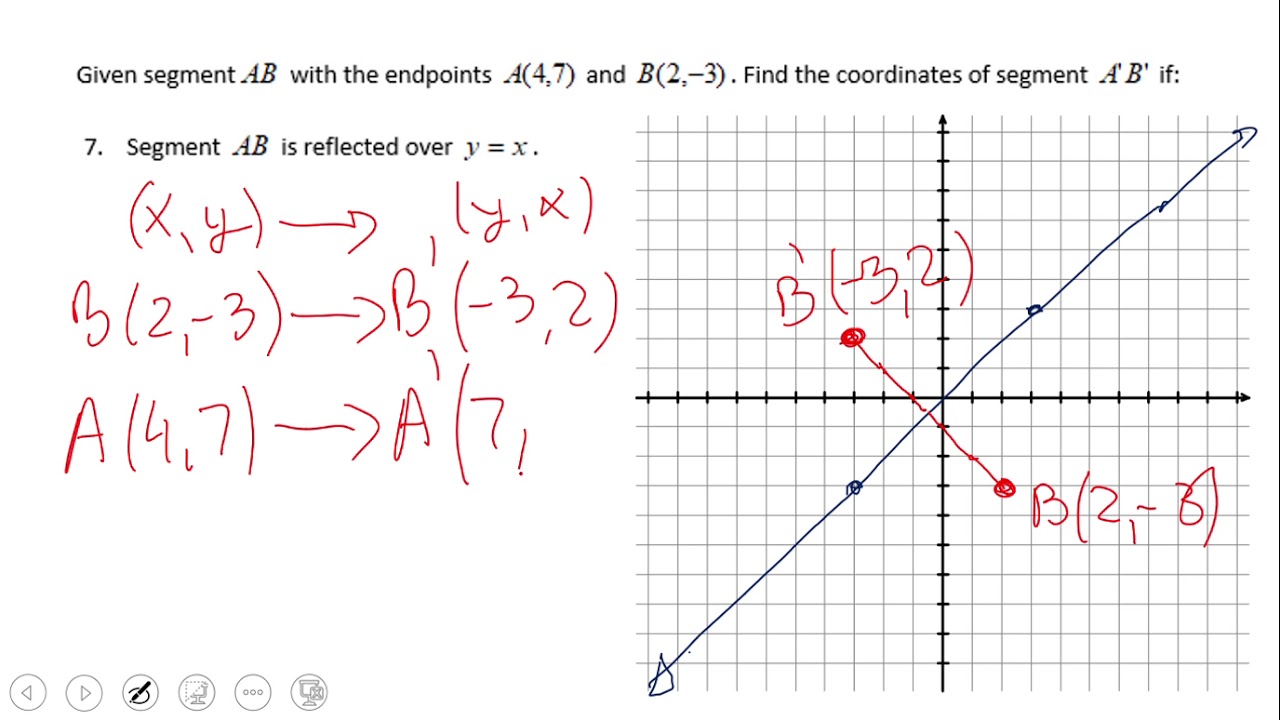
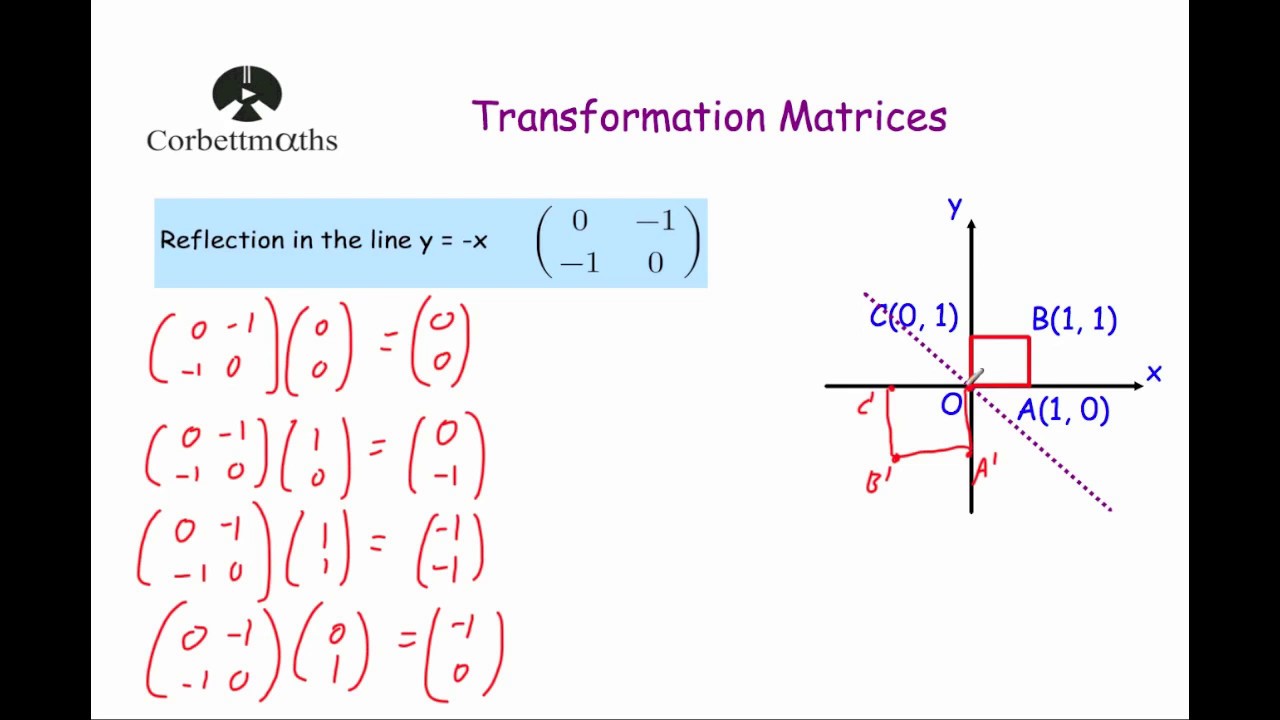
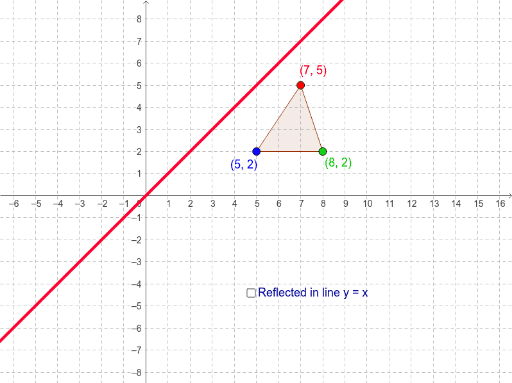
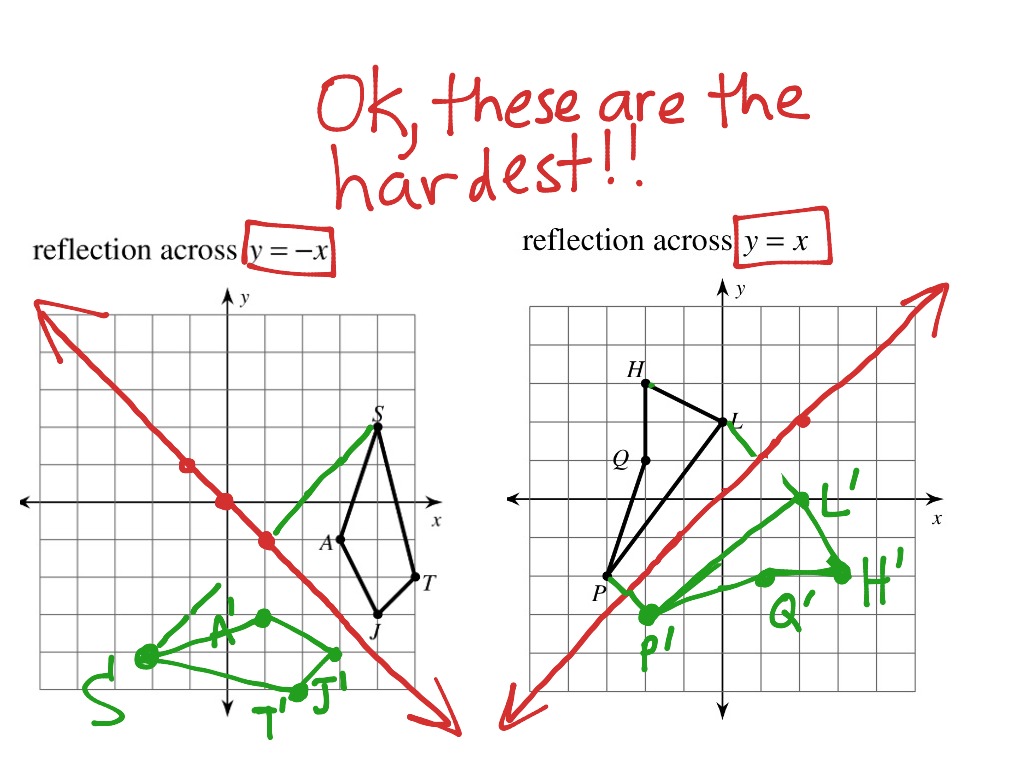
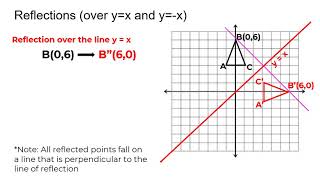
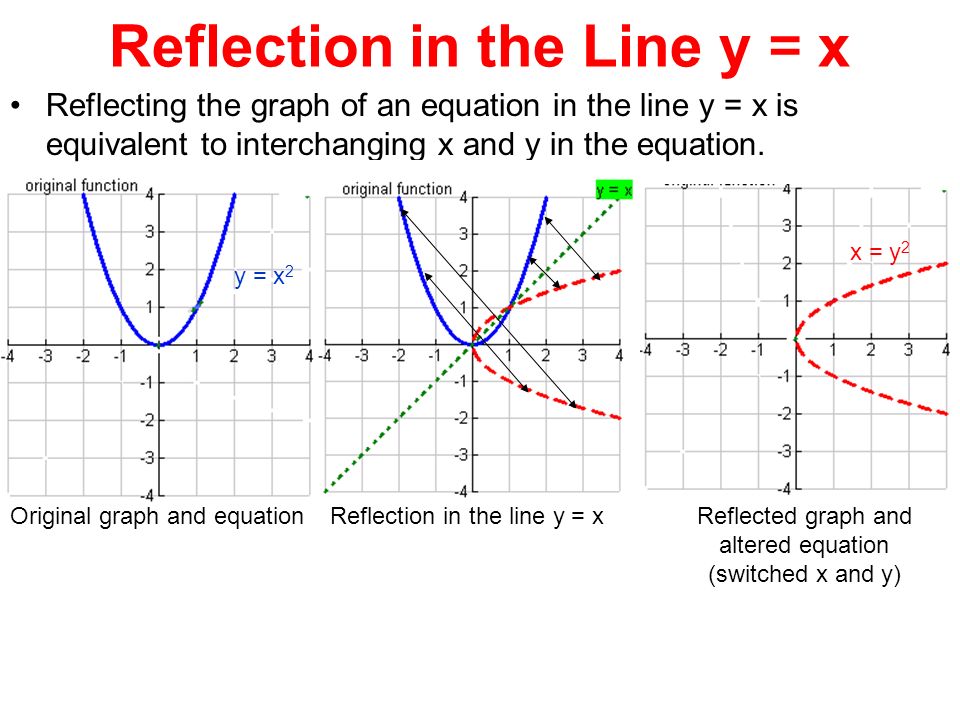
Similarly, when a point is reflected across the line y = x, the xcoordinates and ycoordinates change their place and are negated Therefore, The reflection of the point (x, y) across the line y = x is (y, x) The reflection of the point (x, y) across the line y = – x is (y, x)If you reflect a point across the line y = x, the xcoordinate, and ycoordinate change places If you reflect over the line y =
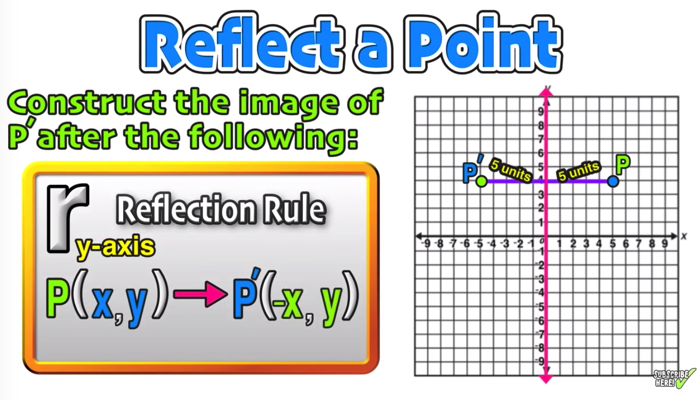
Reflection across y=x formula
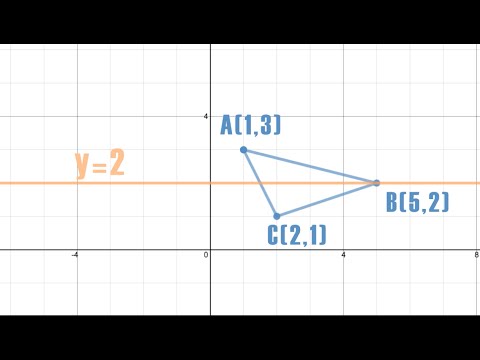
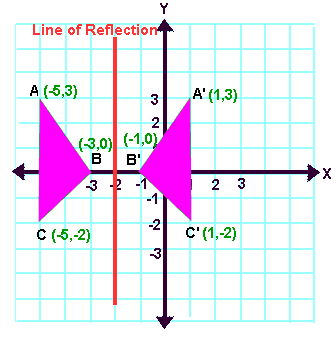
Reflection across y=x formula-Q) and (r, s) (In the graph below, the equation of the line of reflection is y = 2/3x 4 Note that both segments have slopes = 3/2, and the shorter segments on both sides of the line of reflection also have slopes = 3/2 If you are using a xy coordinate axes drawn with a 11 aspect ratio, you can find preimage and image points by just counting From the diagram we see the object point ( − 2, −5) is mapped to (x',y') by a reflection in the line X = 2 we note (1) the ycoordinate is unaffected (2) for reflections the distance from the line of reflection to the object is equal to the distance to the image point ∴ a = 2 2 = 4units so the image point is 4 units from the line of

Reflections Across Y X Geogebra
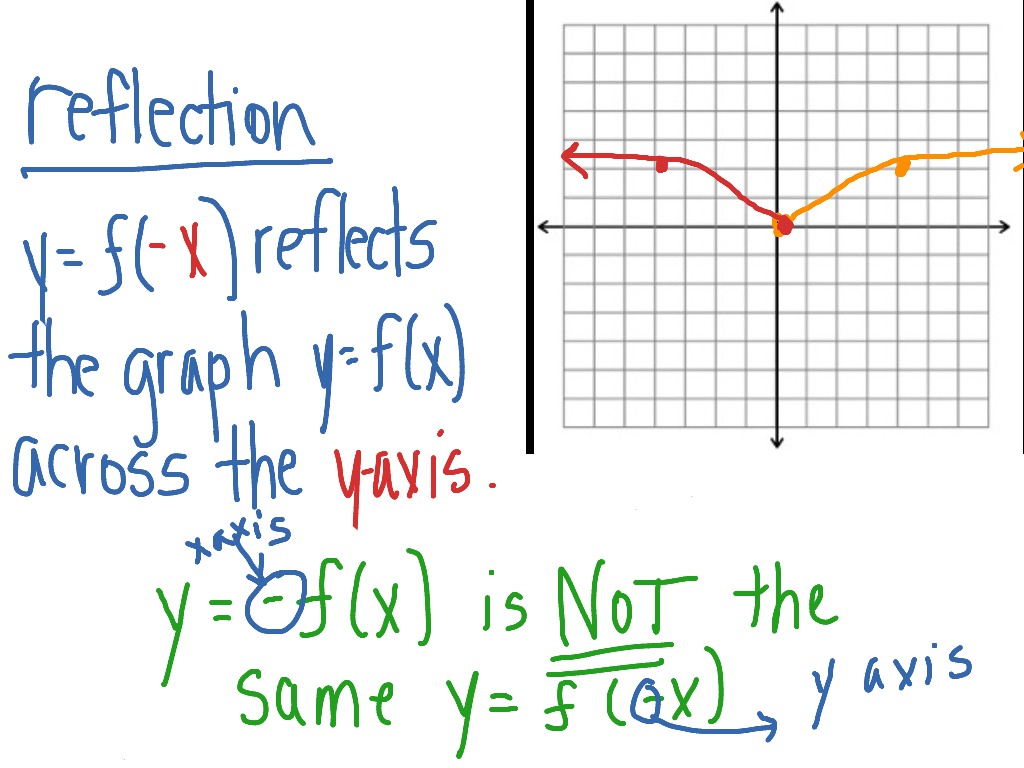
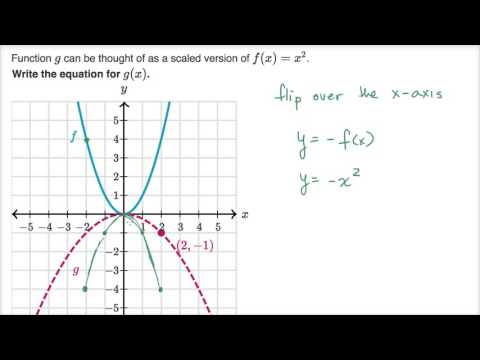
Homework Statement Let T R 2 →R 2, be the matrix operator for reflection across the line L y = x a Find the standard matrix T by finding T(e1) and T(e2) b Find a nonzero vector x such that T(x) = x c Find a vector in the domain of T for which T(x,y) = (3,5) Homework Equations The Attempt at a SolutionThis video is a demonstration of how a reflection can take place across a line where y=x This video is a demonstration of how a reflection can take place across a line where y=x Reflection Across Y=X When reflecting over the line y=x, we switch our x and y, and make both negative Reflection Over Y = X In order to define or describe a reflection, you need the equation of the line of reflection The four most common reflections are defined below
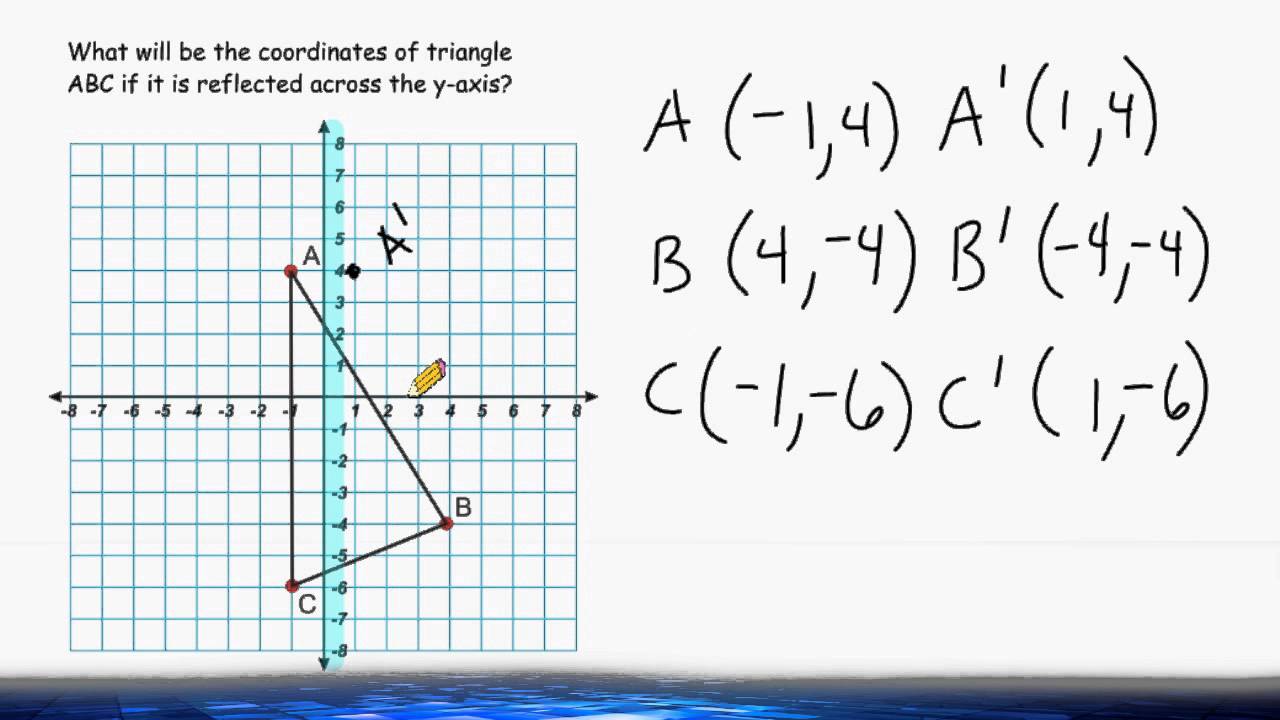
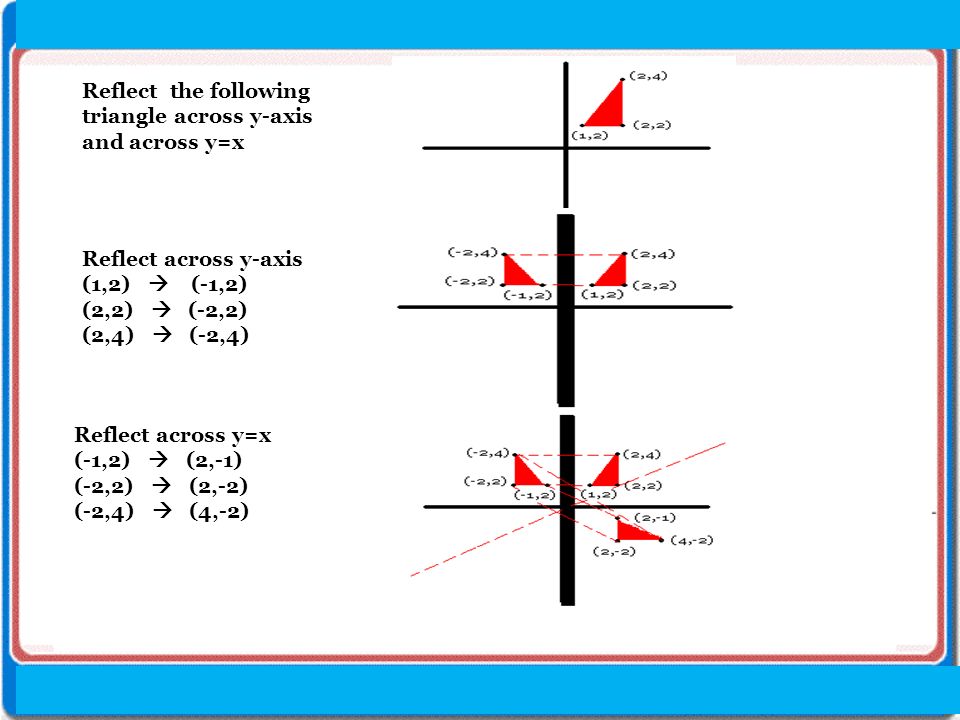
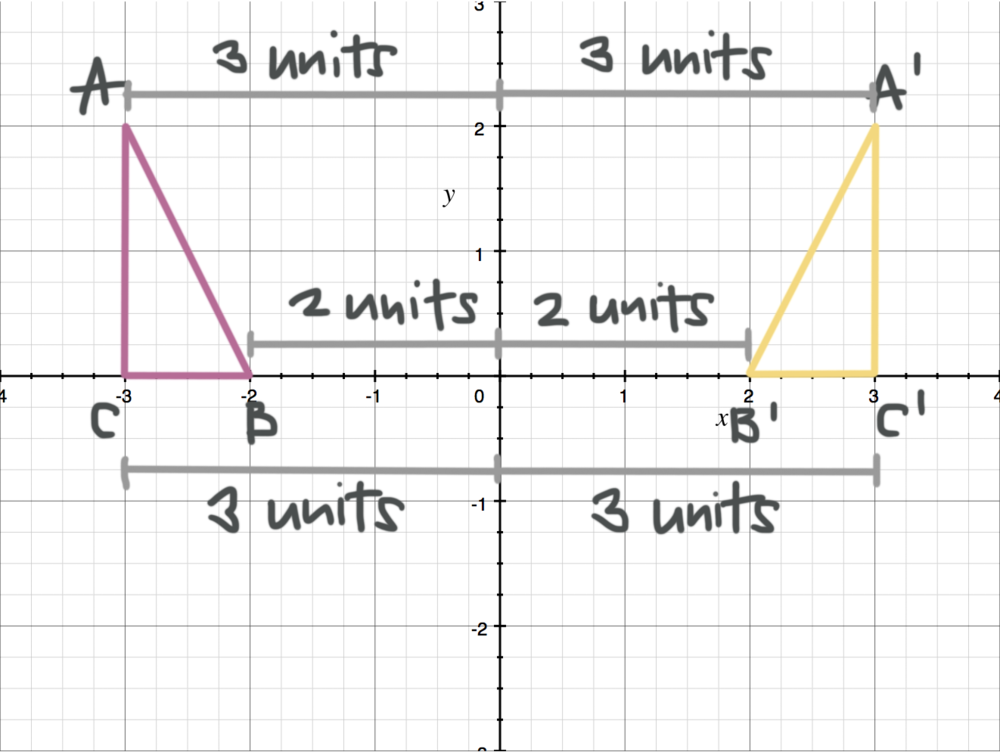
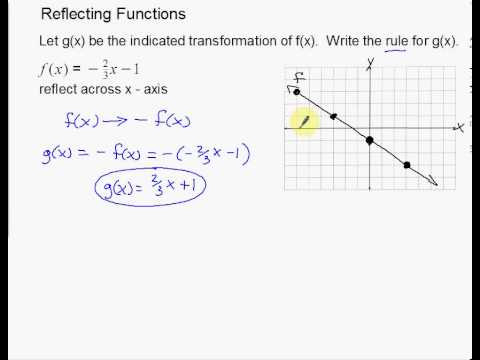
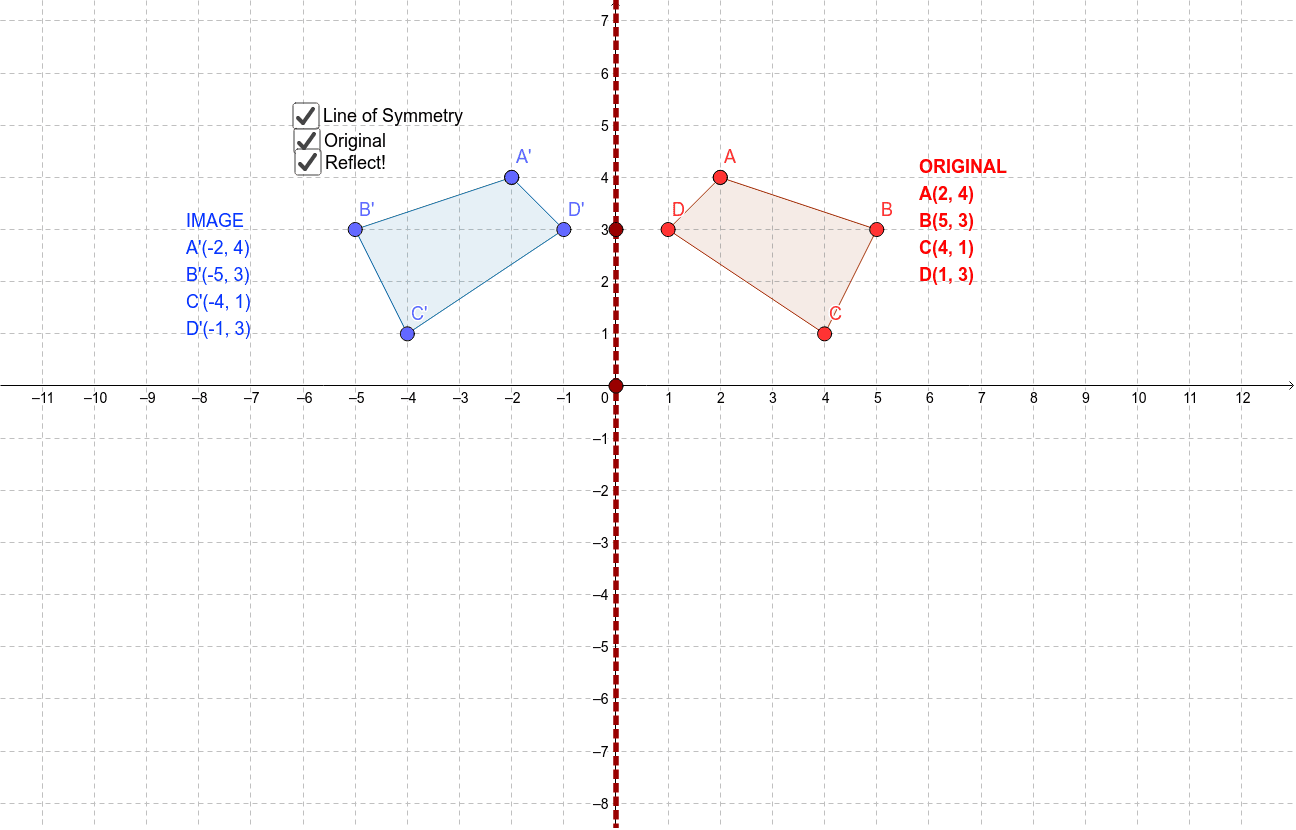
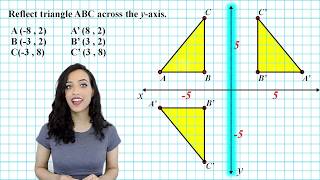
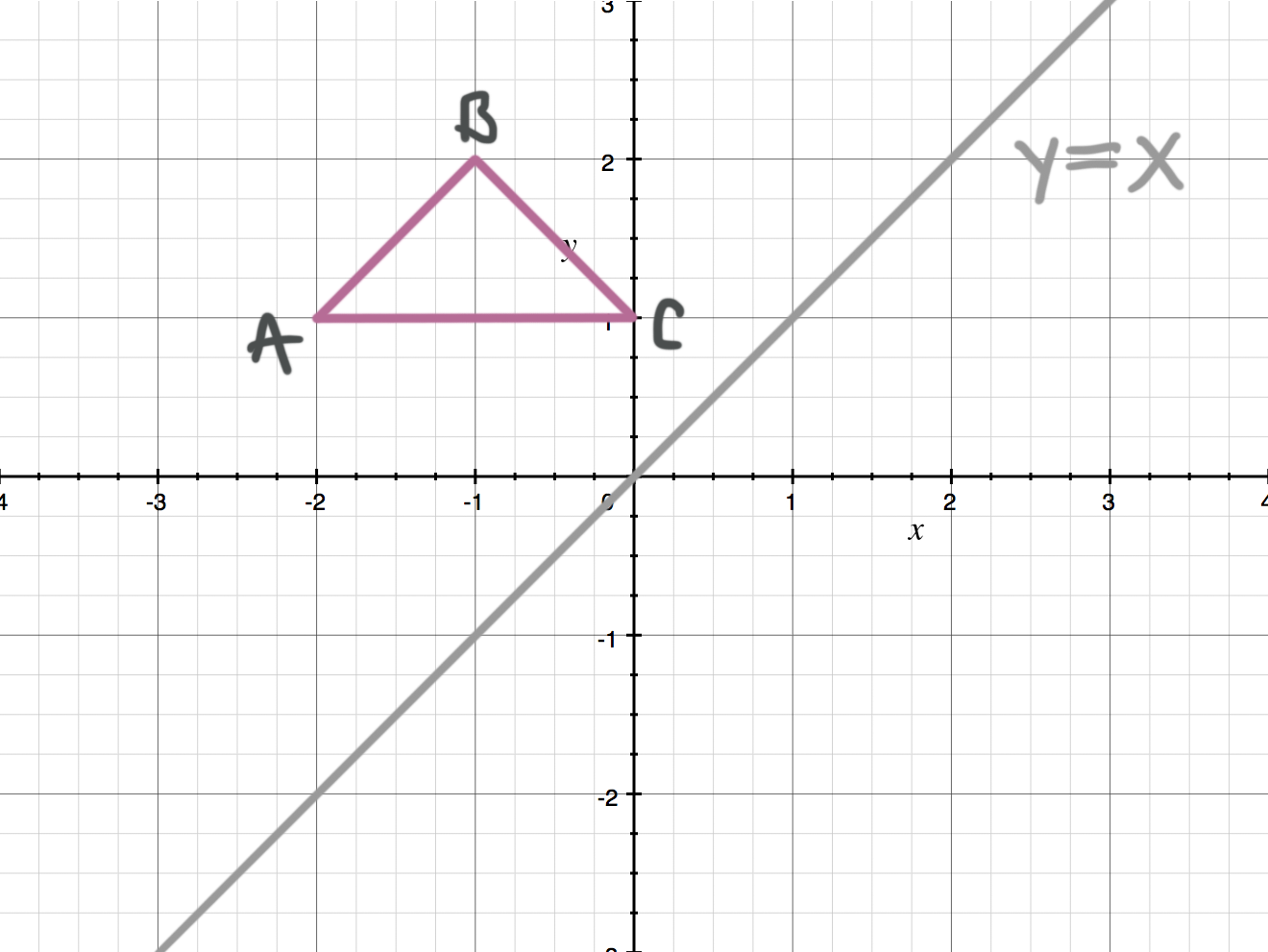
Reflections across the line y = x A reflection across the line y = x switches the x and ycoordinates of all the points in a figure such that (x, y) becomes (y, x) Triangle ABC is reflected across the line y = x to form triangle DEF Triangle ABC has vertices A (2, 2), B (6, 5) and C (3, 6) Triangle DEF has vertices D (2, 2), E (5, 6), and F (6, 3)• Reflection across the yaxis • Shift down 3 units • Vertical scaling by a factor of 4 • Reflection across the xaxis Question Let f(x) = x2 Find a formula for a function g whose graph is obtained from the graph of y = f(x) after the following sequence of transformations • Shift left 4 units • Reflection across the yaxisGiven the function f(x) =x^{4}4x^{3}10 (Figure 135a), find formulas to (a) compress the graph horizontally by a factor of 2 followed by a reflection across the yaxis (Figure 135b) (b) compress the graph vertically by a factor of 2 followed by a reflection across the xaxis (Figure 135c)
Reflection across y=x formulaのギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  | |
 | ||
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 | ||
 |  | |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 | 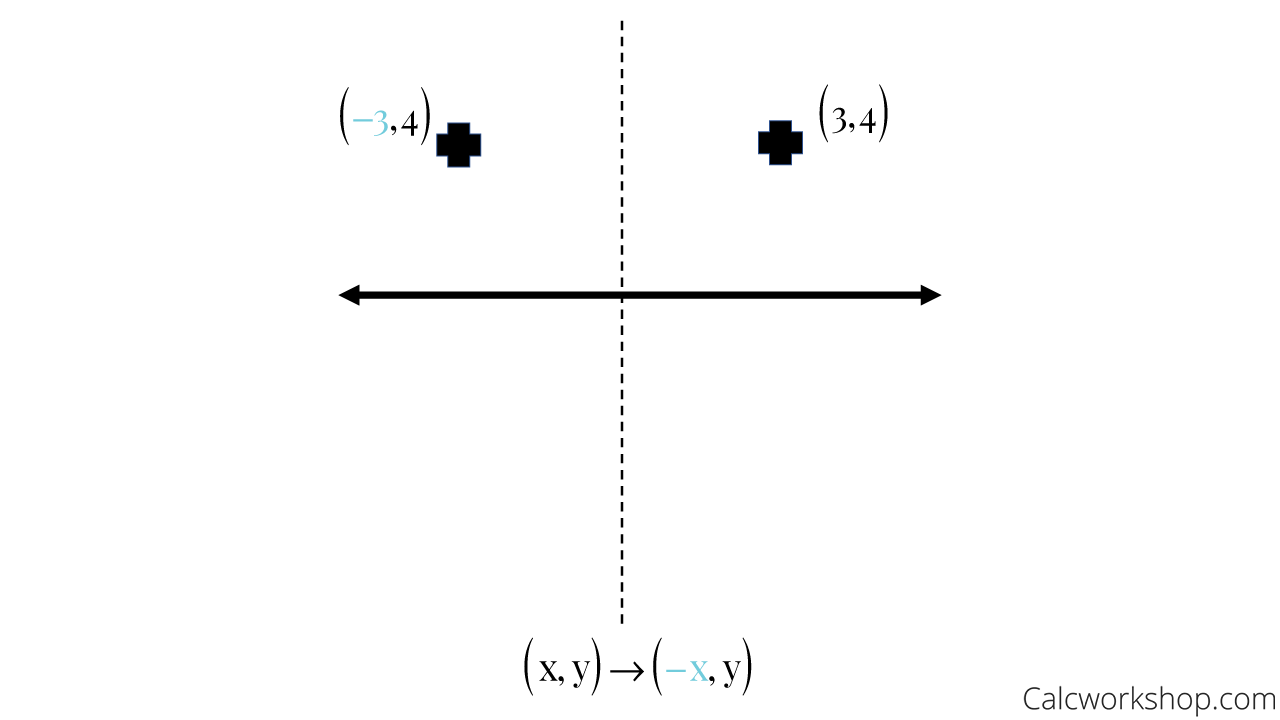 |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 | ||
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |  |  |
 |  | |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  |  |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | 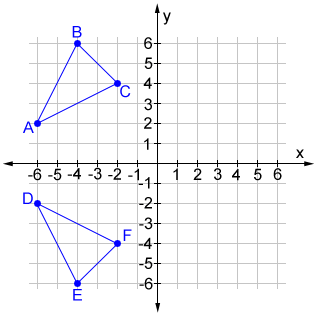 |
「Reflection across y=x formula」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  | |
 |
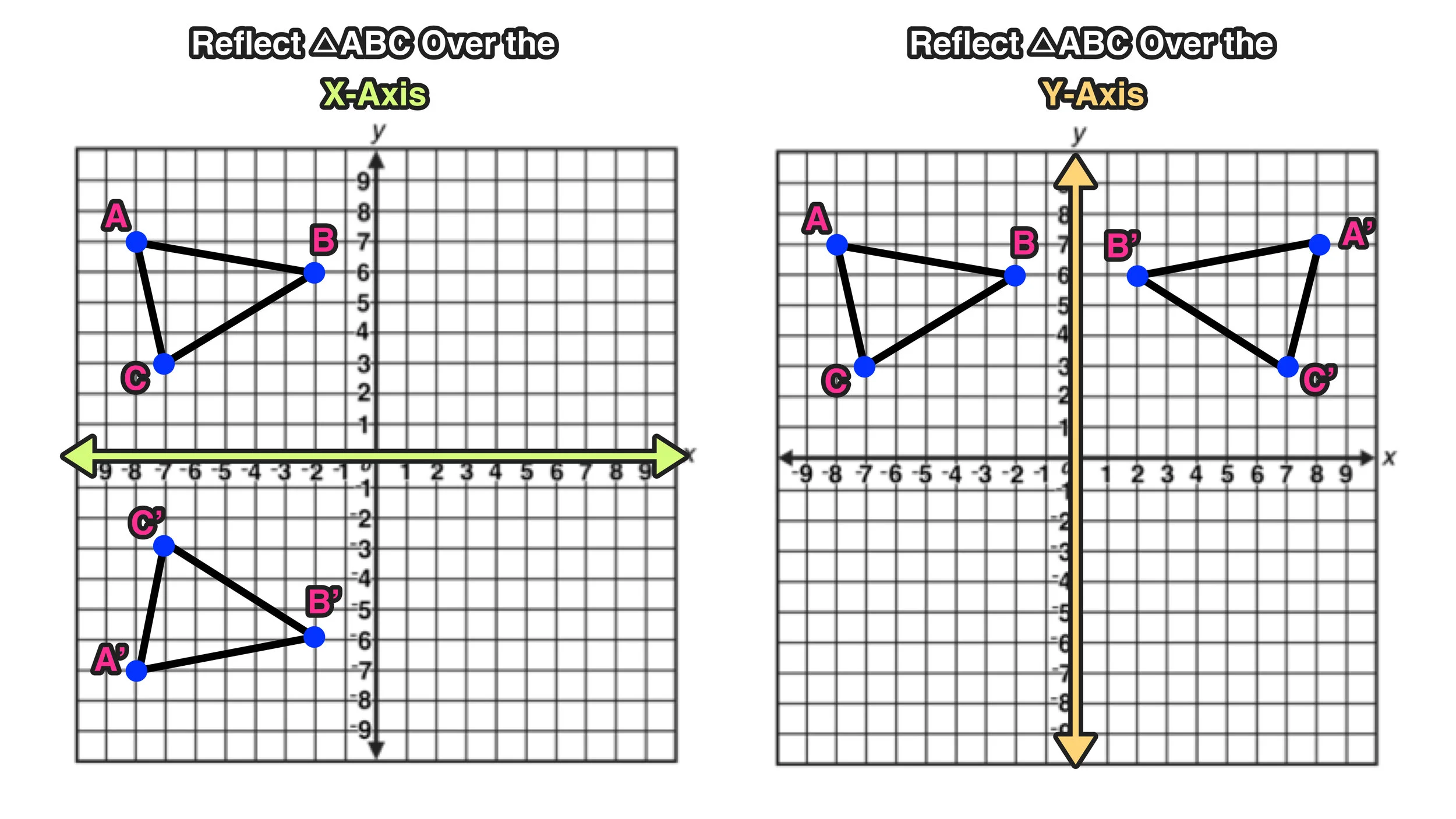
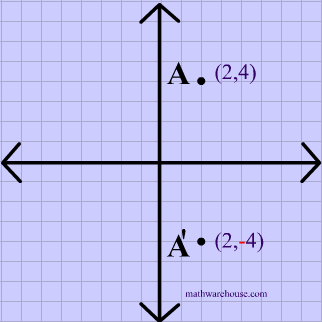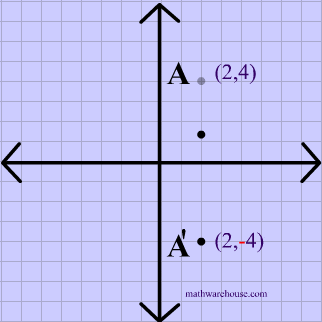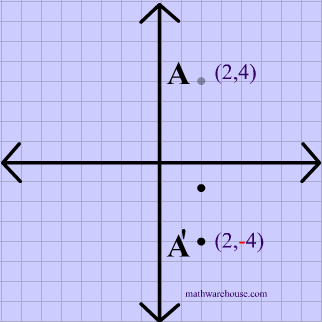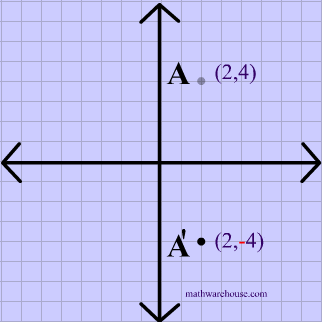
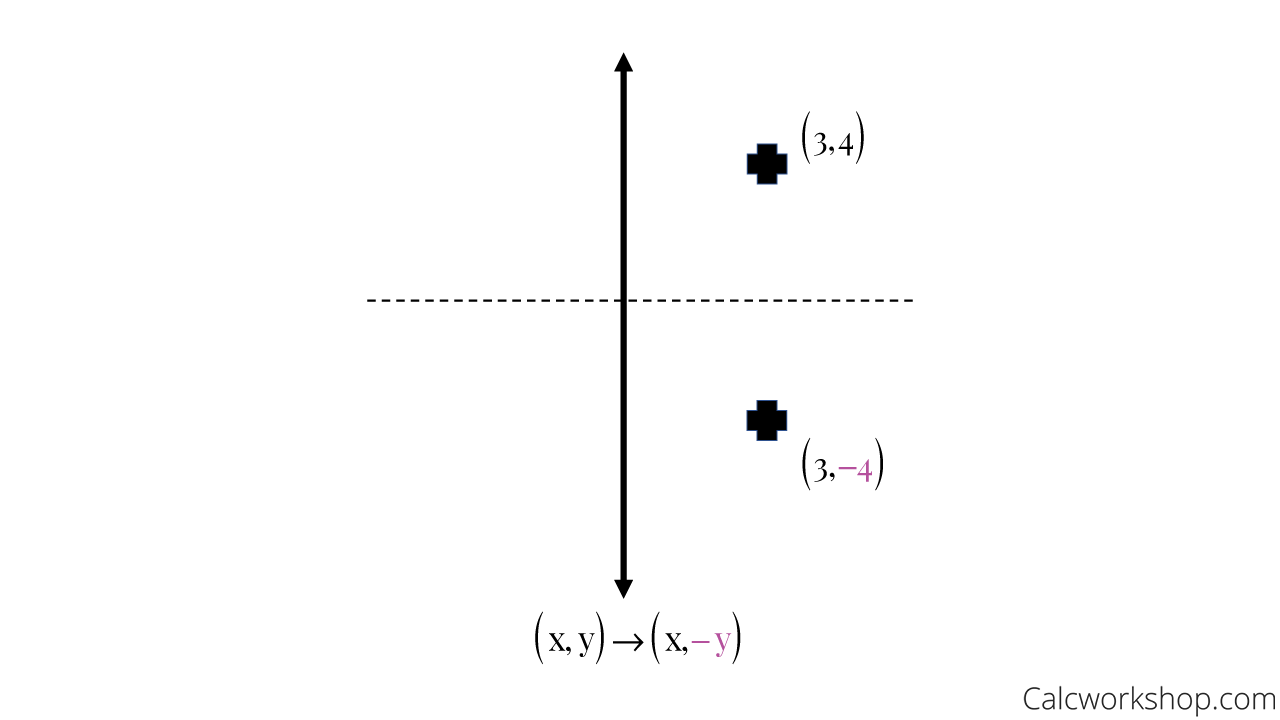
The rule for reflecting over the X axis is to negate the value of the ycoordinate of each point, but leave the xvalue the same Click to see full answer Correspondingly, what is the rule for a reflection across the X axis? And this impression is the reflection of S Show activity on this post Reflecting P (p, q) about L x = a, we get the image at P' (t, q) for some t to be determined Let M = (a, q) be a point on the L and at the same level as P and P' Note that the line L acts as a mirror so that P and P' (at the back of the mirror) are equidistance
Incoming Term: reflection across y=x formula,




0 件のコメント:
コメントを投稿